1/x 3/y=5/2 2/x 3/y=3/2 189874

You can put this solution on YOUR website!Example A circle with center at (3,4) and a radius of 6 Start with (x−a) 2 (y−b) 2 = r 2 Put in (a,b) and r (x−3) 2 (y−4) 2 = 6 2 We can then use our algebra skills to simplify and rearrange that equation, depending on what we need it forFigure 3 The 2area between x = y and y = x − 2 and one horizontal rectangle The height of these rectangles is dy;

点 1 2 を通り 2x 3y 5 0に平行な直線と 垂直な直線の方程式を Clearnote

1/x 3/y=5/2 2/x 3/y=3/2

1/x 3/y=5/2 2/x 3/y=3/2-Simple and best practice solution for 2(x3)5=3(x1) equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework solve 5 / x1 1 / y2 2 and 6 / x1 3 / y2 = 1 0 votes 112k views asked in Class X Maths by priya12 Expert (748k points)

Solving Equations More Than One Step The Algebra

8x2y2/4x3y3 Final result 2x5y5 Step by step solution Step 1 y2 Simplify —— 4 Equation at the end of step 1 y2 ( ( (8 • (x2)) • ——) • x3) • y3 4 Step 2 Equation at the end of step 2 y2 Finding limit of \frac {x^2y^2} {x^3y^3} as (x,y)\to (0,0) duplicate Finding limit of x3y3x2y2On adding (iv) and (v), we get 47u = 94 ⇒ u = 2 ⇒`1/x = 2 ⇒ x = 1/2` On substituting x = 12 in (i), we get `5/ (1⁄2) 3/y = 1` `⇒10 3/y = 1 ⇒ 3/y= (10 – 1) = 9` `y = 3/9 = 1/3` Hence, the required solution is x = `1/2 and y = 1/3` Concept Pair of Linear Equations in Two VariablesAlgebra Graph y=1/2* (x3)^25 y = 1 2 ⋅ (x − 3)2 − 5 y = 1 2 ⋅ ( x 3) 2 5 Find the properties of the given parabola Tap for more steps Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k k a = 1 2 a = 1 2 h = 3 h = 3 k = − 5 k = 5

Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!Add 2 / 3 3 / 5 = 2 5 / 3 5 3 3 / 5 3 = 10 / 15 9 / 15 = 10 9 / 15 = 19 / 15 For adding, subtracting, and comparing fractions, it is suitable to adjust both fractions to a common (equal, identical) denominator The common denominator you can calculate as the least common multiple of both denominators LCM(3, 5) = 15In practice, it is enough to find the common denominatorFirst type the equation 2x3=15 Then type the @ symbol Then type x=6 Try it now 2x3=15 @ x=6 Clickable Demo Try entering 2x3=15 @ x=6 into the text box After you enter the expression, Algebra Calculator will plug x=6 in for the equation 2x3=15 2(6)3 = 15 The calculator prints "True" to let you know that the answer is right More Examples

Y^2 = x^32x^2 WolframAlpha Natural Language Math Input Extended Keyboard ExamplesHere we will look at solving a special class of Differential Equations called First Order Linear Differential Equations First Order They are "First Order" when there is only dy dx, not d 2 y dx 2 or d 3 y dx 3 etc Linear A first order differential equation is linear when it can be made to look like this dy dx P(x)y = Q(x) Where P(x) and Q(x) are functions of x To solve it there is a12 (x 2)^2 (y 3)^2 = 16 13 x^2 (y 4)^2 = 8 14 (x 1)^2 (y 2)^2 = 12 15 (x 6)^2 y^2 = 25 Answer by anantha(86) (Show Source) You can put this solution on YOUR website!

Simultaneous Equations What Are Simultaneous Equations 3221 Let

When Both Linear Equations Of A System Are

1 Which value is a solution of the equation 5 4x = 3 0 2 3 1/4 2 Which ordered pair is a solution of the equation y = x 4 (2, 6) (6, 2) (2, 6) (3, 1) 3 Which ordered pair is a solution of the equation y = 3x (2, 9)If you FOIL the last 2 factors (x5)(x2) you get Now distribute a 3 to every term So the right choice is a) You can always let x and y be any numbers to verify this, or you can graph these and they should be equal1·2 x3 1·2·3 ·• For large x > 0, ex > x p p!

Solved Find The Derivative 1 Y 5 2 X 3 2 F X 9t 2 2 3 Chegg Com

1 The Region R Is The Region Bounded By The Functions Y X 3 And X 1 Y 2 Find The Volume Homeworklib

Add 2 / 3 3 / 5 = 2 5 / 3 5 3 3 / 5 3 = 10 / 15 9 / 15 = 10 9 / 15 = 19 / 15 For adding, subtracting, and comparing fractions, it is suitable to adjust both fractions to a common (equal, identical) denominator The common denominator you can calculate as the least common multiple of both denominators LCM(3, 5) = 15In practice, it is enough to find the common denominator1 0 f(x;y) 2 The total probability is 1 We now express this as a double integral Z d Z b f(x;y)dxdy = 1 c a Note as with the pdf of a single random variable, the joint pdf f(x;y) can take values greater than 1;We get their width by subtracting the xcoordinate of the edge on the left curve from the xcoordinate of the edge on

Solved Evaluate An Expression Evaluate X 3 Y 2 For X Chegg Com

Solving Equations More Than One Step The Algebra

3x(x 2 2x – 5) Content Continues Below Factor 26 x sqrt9 y 3 – 13 y sqrt4 x 2 y xy sqrt169 x 4 y 3 , assuming that all variables are nonnegativeSOLUTION 1 Begin with x 3 y 3 = 4 Differentiate both sides of the equation, getting D ( x 3 y 3) = D ( 4 ) , D ( x 3) D ( y 3) = D ( 4 ) , (Remember to use the chain rule on D ( y 3) ) 3x 2 3y 2 y' = 0 , so that (Now solve for y' ) 3y 2 y' = 3x 2, and Click HERE to return to the list of problems SOLUTION 2 Begin with (xy) 2 = x y 1 Differentiate both sidesSolve the given inequalities x – 2y ≤ 2, x y ≥ 3, –2x y ≤ 4, x ≥ 0, y ≥ 0 graphically in two – dimensional plane asked Jul 22 in Linear Equations by KumarArun ( 145k points) pair of linear equations in two variables

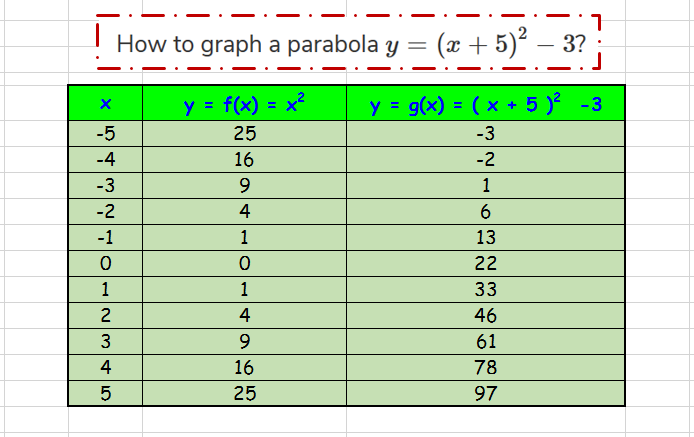
How To Graph A Parabola Y X 5 2 3 Socratic

Solve The Following Simultaneous Equations Graphically 1 2x 3y 12 X Y 1 2 X 3y 1 3x Brainly In

 From equation (2) y = 3 2 z Consider equation (1) Substitute for y giving x y = 5 2 → x 3 2z = 5 2 2 3 x z = 5 2 x z = 3 2 × 5 2 = 15 4 Answer link EZ as piAnswer (1 of 8) Given 2^x=3^y=6^z Lets assume that each and every term is equal to k Which implies 2^x=k Now by applying logarithm on both sides we get x=log k to base 2 and 1/x= log 2 to base k And 3^y=k Again by applying logarithm on both sides we get y=log kSolution We first calculate the partial derivatives at the point in question

Solve For X And Y 40 X Y 2 X Y 5 And 25 X Y 3 X Y 1 Mathematics Topperlearning Com Idbi1itt

Vectors And The Geometry Of Space Pagina 2 Monografias Com

SOLUTION 1 Begin with x 3 y 3 = 4 Differentiate both sides of the equation, getting D ( x 3 y 3) = D ( 4 ) , D ( x 3) D ( y 3) = D ( 4 ) , (Remember to use the chain rule on D ( y 3) ) 3x 2 3y 2 y' = 0 , so that (Now solve for y' ) 3y 2 y' = 3x 2, and Click HERE to return to the list of problems SOLUTION 2 Begin with (xy) 2 = x y 1 Differentiate both sides if sin x = 1/3 and sec y = 5/4 , where x and y lie between 0 and π/2, evaluate sin(x y) So we know that the first number is the xaxis (2) and the second one the yaxis (6) and the linear equation is y= x4 let's plug in the numbers 6=24 Wait does that make sense to you The answer is A (1,5) y=x4 5= 14 Hope it make and that was a fake mssue 👍

Solve This Problem 5 X 1 1 Y 2 2 And 6 X 1 3 Y 2 1 Brainly In

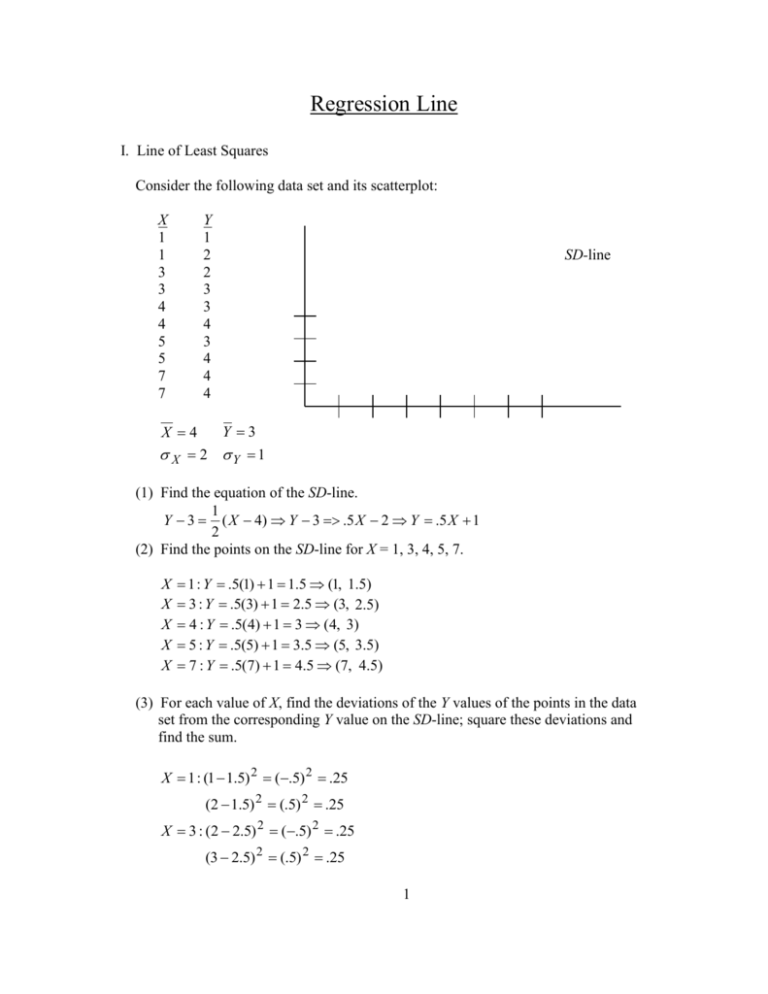
Regression Line

X^2(y(x^2)^(1/3))^2 = 1 Natural Language; 1/2a 5/3 = 3/2 1/2a = 5/3 3/2 1/2a = 1/6 (taking LCM) Hence a = 3 Now we know a = x 2y = 3 (v) and b = 3x 2y = 1 (vi) Now solving by normal pair of linear eqns in two vars, adding (v) and (vi) we get 4x = 3 1 x = 2/4 = 1/2 Substituting in (v), 1/2 2y = 3 (1 4y)/2 = 3 (LCM) 1 4y = 6 So y = (61)/4 = 5/4Sol the equation is of the form (xh)^2(yk)^2=r^2 then the center=(h,k) and radius=r 1) (x2)^2(y3)^2=16 comparing with (xh)^2(yk)^2=r2 here h=2,k

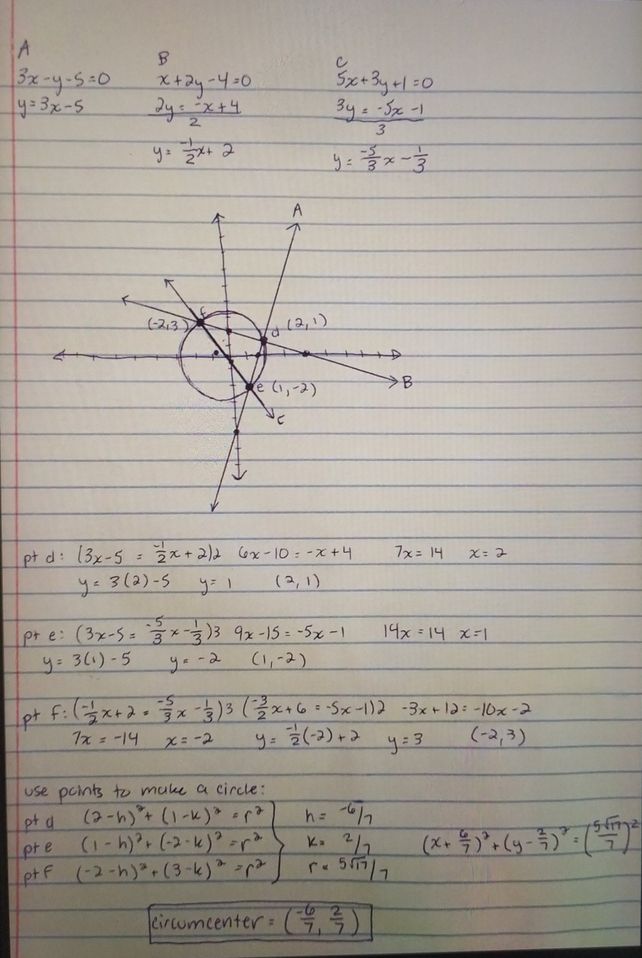
1 Find The Circumcentre Of Th See How To Solve It At Qanda

Chapter 6 Simultaneous Linear Equations Including Problems Selina Concise Mathematics Part I Solutions For Class 9 Mathematics Icse Topperlearning

P 330 (3/23/08) Section 145, Directional derivatives and gradient vectors Example 2 What is the derivative of f(x,y) = x2y5 at P = (3,1) in the direction toward Q = (4,−3)?Y=3 (x5) (x2) Simple and best practice solution for y=3 (x5) (x2) equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve it  Y=3x1 for x=1, 2, 3, 4, 5, 6 Answered by a verified Math Tutor or Teacher Disclaimer Information in questions, answers, and

Solved 1 10 Y 2 5 1 5 Y 3 10 X 3 5 2 X 5 6 Chegg Com

Bachelor Of Information Technology Introductory Mathematics En 11

 Transcript Example 18 Solve the following pair of equations by reducing them to a pair of linear equations 5/(𝑥 −1) 1/(𝑦 −2) = 2 6/(𝑥 −1) – 3/(𝑦 −2) = 1 5/(𝑥 − 1) 1/(𝑦 − 2) = 2 6/(𝑥 − 1) – 3/(𝑦 − 2) = 1 So, our equations become 5u v = 2 6u – 3v = 1 Thus, our equations are 5u v = 2 (3) 6u – 3v = 1 (4) From (3) 5u v = 2 v = 2 So, the integrand can be simplified as √1 ( dx dy)2 = √1 (y − 1)1 22 = √y = y1 2 Finally, we have L = ∫ 4 0 y1 2dy = 2 3y3 24 0 = 2 3 (4)3 2 − 2 3(0)3 2 = 16 3 Hence, the arc length is 16 3 I hope that this helps Answer link If √x √y = 5, then dy/dx at (4,9) is (a) 2/3 (b) 3/2 (c) 3/2 (d) 2/3 asked in Mathematics by KumariMuskan ( 339k points) bseb model set

Non Linear Systems

6 1 Tables And Graphs Simultaneous Linear Equations Siyavula

⇒ ex xn > x −n p!Select a few x x values, and plug them into the equation to find the corresponding y y values The x x values should be selected around the vertex Tap for more steps Replace the variable x x with 4 4 in the expression f ( 4) = 3 ( 4) 2 − 30 ⋅ 4 76 f ( 4) = 3 ( 4) 2 30 ⋅ 4 76 Simplify the resultSubtract 3 y from both sides 2x=53y 2 x = 5 − 3 y Divide both sides by 2 Divide both sides by 2 \frac {2x} {2}=\frac {53y} {2} 2 2 x = 2 5 − 3 y Dividing by 2 undoes the multiplication by 2 Dividing by 2 undoes the multiplication by 2

If A 1 2 5 1 1 1 2 3 1 Find A 1 Hence Solve The Following System Of Equations X 2y 5z 10 X Y Z 2 2x 3y Z 11 Sarthaks Econnect Largest Online Education Community

Given Z F X Y X X U V Y Y U V With X 5 2 3 Y 5 2 1 Calculate Z U 5 2 In Terms Of Some Of The Values Given In The Table Below F X 5 2 A F Y 5 2 2 X U 5 2

Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, musicAbout Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us CreatorsClick here👆to get an answer to your question ️ If (1, 2), (4, y), (x, 6) and (3, 5) are the vertices of a parallelogram taken in order, find x and y

Ml Aggarwal Solutions For Class 9 Maths Chapter 5 Simultaneous Linear Equations

1 Variables Evaluation

For three variables, a level set is typically a surface, called a level surface EXAMPLE 1415 Suppose the temperature at (x,y,z) is T(x,y,z) = e−(x2y2z2) This function has a maximum value of 1 at the origin, and tends to 0 in all directions2 1 2 1 1 x y=1−x y x y support set Blue subset of support set with y>1−x (a) We find c by setting 1 = Z ∞ −∞ Z ∞ −∞ f(x,y)dydx = Z 1 0 Z 2 0 (cx2 xy 3)dydx = 2c 3 1 3, so c = 1 (b) Draw a picture of the support set (a 1by2 rectangle), and intersect it with the set {(x,y) x y ≥ 1}, which is the region above the Ex 21, 1 If (x/3 " 1, y –" 2/3) = (5/3 "," 1/3) , find the values of x and y (x/3 " 1, y –" 2/3) = (5/3 "," 1/3) Since the ordered pairs are equal, corresponding elements are equal Hence x/3 1 = 5/3 x/3 = 5/3 – 1 x/3 = 2/3 x = 2 y – 2/3 = 1/3

4x Y 3 8 3 And X 2 3y 4 5 2 Solve By Elimination Method Maths Pair Of Linear Equations In Two Variables Meritnation Com

Maths Class 10 Pages 51 100 Flip Pdf Download Fliphtml5

352 Chapter 14 Partial Differentiation k;Respuesta de 52 (1x)=2x3 Sumamos todos los números y todas las variables Nos deshacemos de los paréntesis Sumamos todos los números y todas las variables No hay solución para esta ecuación El resultado de la ecuación 52 (1x)=2x3 para usar en su tarea domésticaIn general this is called a level set;

Ex 2 3 9 Solve 2y 5 3 26 3 Y Chapter 2 Ncert Maths

Vectors And The Geometry Of Space Monografias Com

⇒ sin − 1 x 3 − sin − 1 y 3 = 2 cot − 1 a Differentiating both side, we get 1 − x 6 1 d x d ( x 3 ) − 1 − y 6 1 d x d ( y 3 ) = 0This integral of a function along a curve C is often written in abbreviated form as ∫ C f ( x, y) d s Example 1621 Compute ∫ C y e x d s where C is the line segment from ( 1, 2) to ( 4, 7) We write the line segment as a vector function r = 1, 2 t 3, 5 ,Find X And Y, If `2x 3y = (2,3),(4,0) and 3x 2y = (2, 1),(1,5)`

1 はできたんですけど 2 が模範解答を見てもわかりません Clearnote

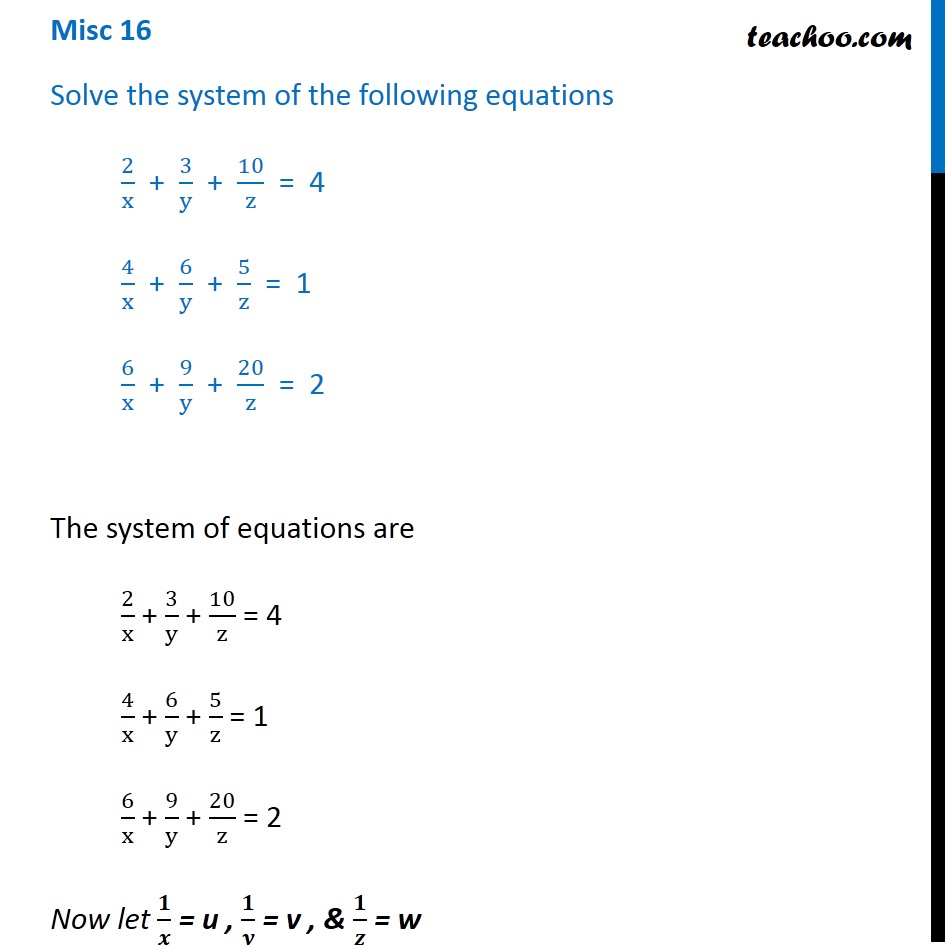
Misc 16 Solve Equations 2 X 3 Y 10 Z 4 4 X 6 Y 5 Z 1

It is a probability density, not a probability In 1805 we won't expect you to be experts at double integration Here's\log _2(x1)=\log _3(27) 3^x=9^{x5} equationcalculator y=2x^{2}5x3 en Related Symbolab blog posts High School Math Solutions – Quadratic Equations Calculator, Part 1 Best Answer #2 299 10 The distance formula (actually, the Pythagorean Theorem) that gives the distance between the points (x 1, y 1) and (x 2, y 2) is d = √ (x 2 x 1)2 (y 2 y 1) 2 The distance from x (1, 3) and y (3,0) call x 1 = 1, y 1 = 3, x 2 = 3, y 2 = 0

If 2 X 3y 12 And 3 X 2y 5 Then Youtube

Graphing Parabolas

• For p > n, lim x→∞ xp−n = ∞, then lim x→∞ ex xn = ∞ Quiz Quiz 1 domain of ln 1x2 (a) x > 1, (b) x > −1, (c) any x 2 domain of ln x p 4x2 (a) x 6= 0, (b) x > 0, (c) any x 2 Differentiation and Graphing 21 Chain Rule Differentiation

Using Matrix Method Solve The System Of Equations 3x 2y 2z 3 X 2y 3z 6 2x Y Z 2 Sarthaks Econnect Largest Online Education Community

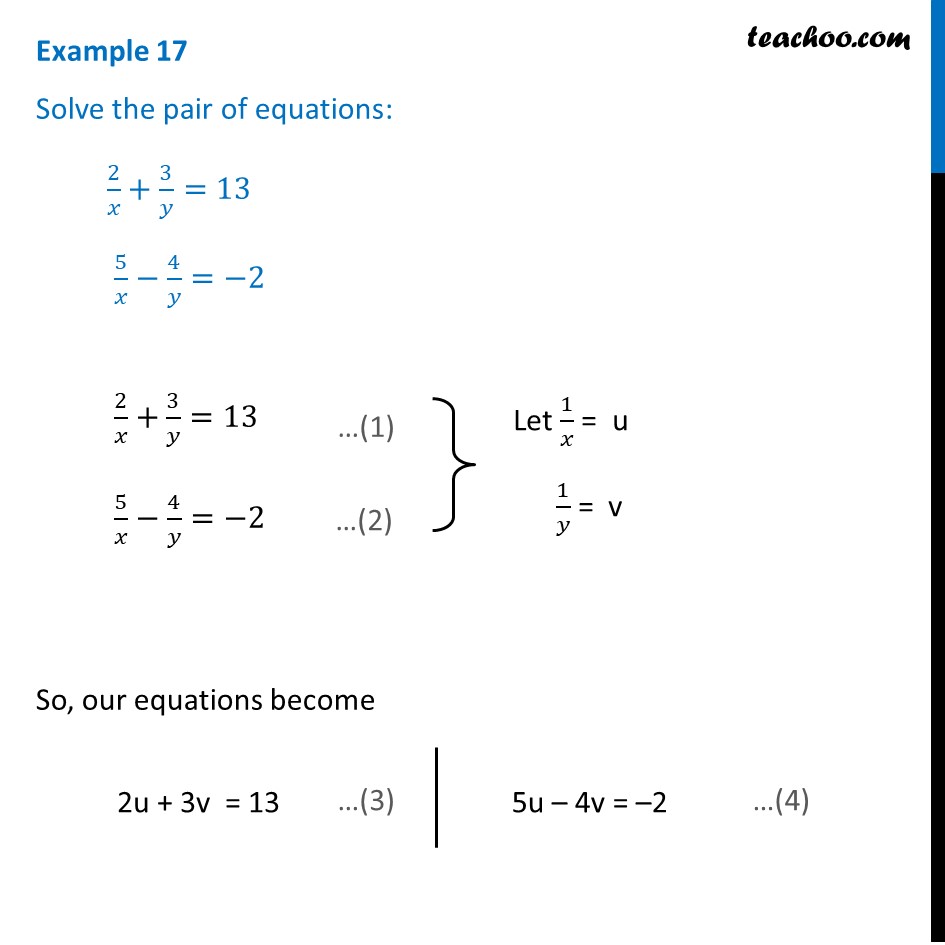
Example 17 Solve 2 X 3 Y 13 5 X 4 Y 2 Examples

Ppt Warm Up Factor Completely Powerpoint Presentation Free Download Id

Solved Solve 3x 2y 10 3 5x 3y 15 2x 4y 6 4 X 2y 3 3x Chegg Com

Math 50 Final Practice D 5 2 X 3 4 3 1

2

2

Find The Circumcenter Whose Sides Are 3x Y 5 0 X 2y 4 0 5x 3y 1 0 Quora

Let F R Arrow R Be Defined As F X Begincases 55x Te

Related Videos Of If A 2 3 5 3 2 4 1 1 2 Find A 1 Use It To Solve The System Of Equations 2x 3y 5z 11 3x 2y 4z 5 And X Y 2z 3

Regents Practice Test 3

3 X 1 Y 9 And 2 X 3 Y 5 By Elimination Method Brainly In

Relaciones Binarias Pdf

For A Pair Of Equations 2x 3y 10 And 3x Y 4 Find X

Thomasborer Ch

1 X 3 Y 5 2 2 X 3 Y 3 2 Solve The Equation Brainly In

点 1 2 を通り 2x 3y 5 0に平行な直線と 垂直な直線の方程式を Clearnote

Functions And Their Graphs Quadratic Function Field

Solved Given 2x 3y Z 1 3x 5y 2z 8 X 2y Chegg Com

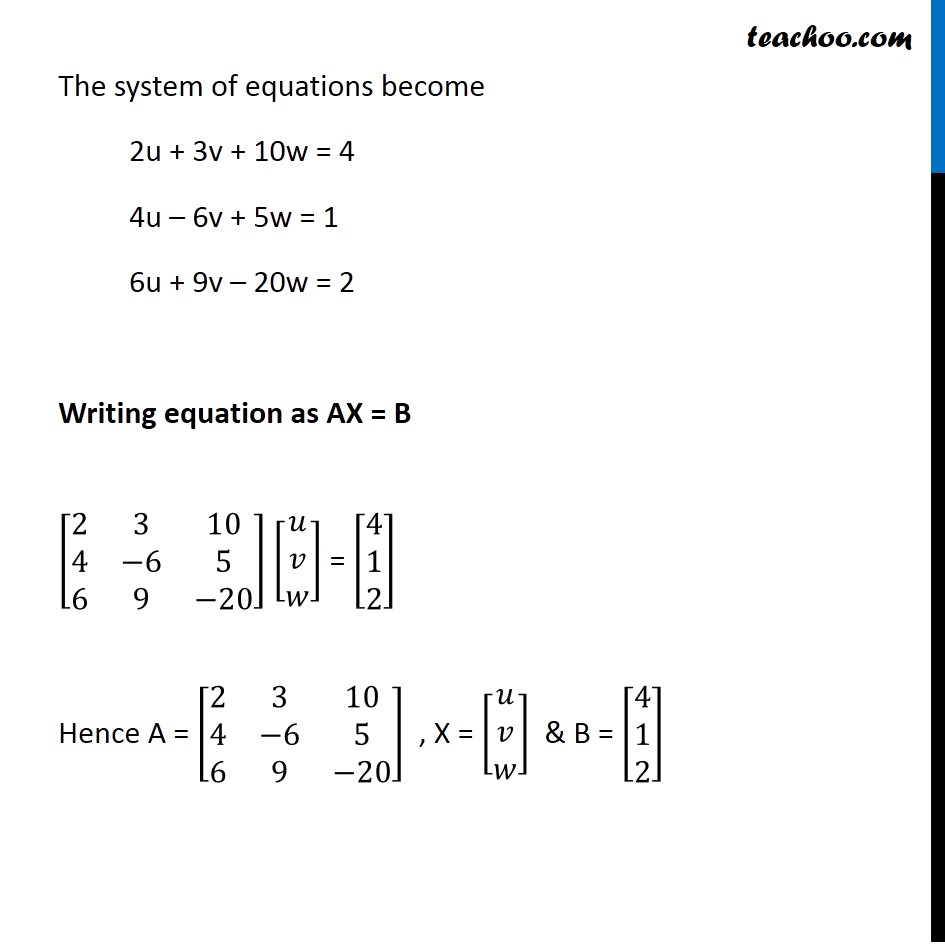
Misc 16 Solve Equations 2 X 3 Y 10 Z 4 4 X 6 Y 5 Z 1

B Find The Vertex Of Each Of The Following Quadrat Gauthmath

X 3 2 Y 5 2 49 By Jodi Ann Bradley

O1ungeorxskx M

How To Solve Using The Gauss Elimination Method Y Z 2 2x 3z 5 X Y Z 3 Quora

Linear Equations Find The Domain And The Range Of The Following Set 4 5 7 8 1 3 3 3 2 3 Pdf Free Download

500 Quadratic Equation Questions For Ibps 1 500 Quadratic Equation Questions

3x 2y 5 2 And 1 3 3y 4 3 Problem Set 1 Q5 3 Linear Equation In Two Variables Youtube

Find A So That 3 A Lies On The Line Represented By 2x 3 Scholr

2

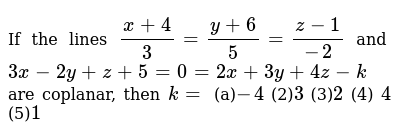
If The Lines X 4 3 Y 6 5 Z 1 2 And 3x 2y Z 5 0 2x 3y 4z K Are Coplanar Then K A 4 2 3 3 2 4 4 5 1

Simultaneous Equations 1 Ppt Download

Mathematics Class 9th Chapter 4 Solution

Using Matrices Solve The Following System Of Equations 2x 3y 5z 11 3x 2y 4z 5 X Y 2z 3 Quora

D 3 F Xy X Y 1 G 2 X 3 Y 3 2 Which Is Positive On The Download Scientific Diagram

Class 12 Ncert Math Solution

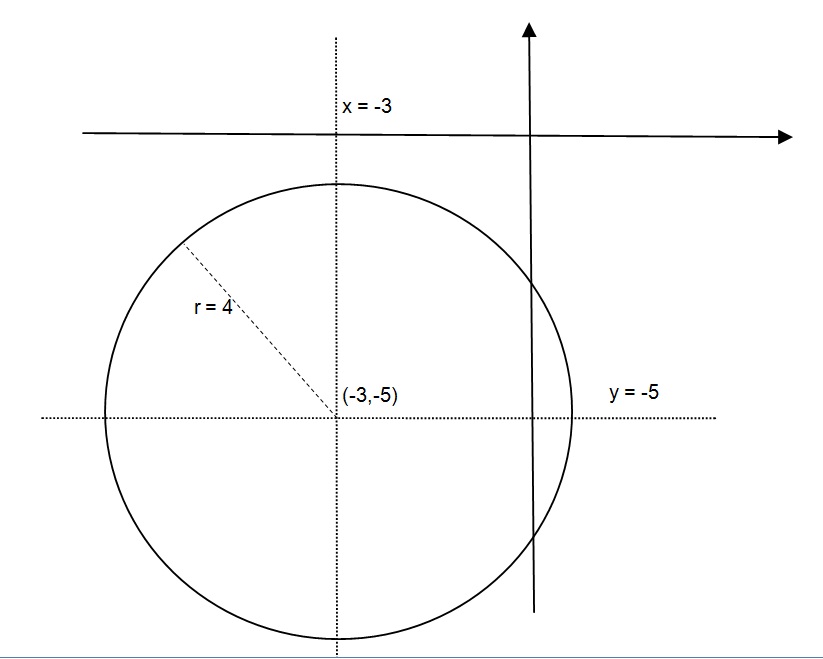
How Do You Graph X 3 2 Y 5 2 16 Example

If X 3 2 Y 5 3 4 3 1 3 Find The Value Of X And Y Answer It Now Maths Sets 1375 Meritnation Com

Chapter 2 Polynomial Factoring 2 1 Factoring Out The Gcf

Finding Linear Equations

Solved Use Logarithmic Differentiation To Find The Derivative Of Y X 3 2 X 2 5 X 4 7 4 Find The Equation Of The Tangent Line To The Function Course Hero

12 X Y 2 Quad 3 X 2 Y 5 2 X Y 5 Quad 3 X 5 Y 3 X 3 Y 4 Quad 4 X Y 3 2 X Y 2 Quad 3 X 4 Y 4 X 3 Y 1 2 X Y B Inversion Method

Elimination Method Day 1 Ppt Download

Ap8vcui 423lvm

Exercise 5 3 Straight Line Problem Questions With Answer Solution Mathematics

Ncert Solutions For Class 9 Maths Chapter 4 Linear Equations In Two Variables

Find The Points On The Line X 2 3 Y 1 2 Z 3 2 At A Distance Of 5 Units From The Point P 1 3 3 Sarthaks Econnect Largest Online Education Community

What Is Y 1 5 4 X 2 Written Using The Standard Form Of The Equation Of A Line Socratic

Warm Up Determine If 3 4 Is A Solution To The Equation 1 Y 2x 2 2 6x 3y 6 4 2 3 2 4 6 2 6 3 3 4 6 18 12 6 Yes Ppt Download

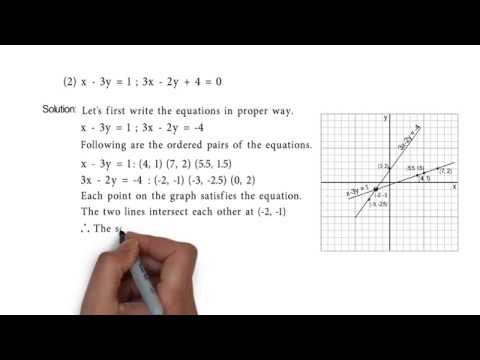
X 3y 1 And 3x 2y 4 0 Of Problem Set 1 Q3 2 In Linear Equation In Two Variables Of Algebra Youtube

Wolfram Alpha Examples Step By Step Solutions

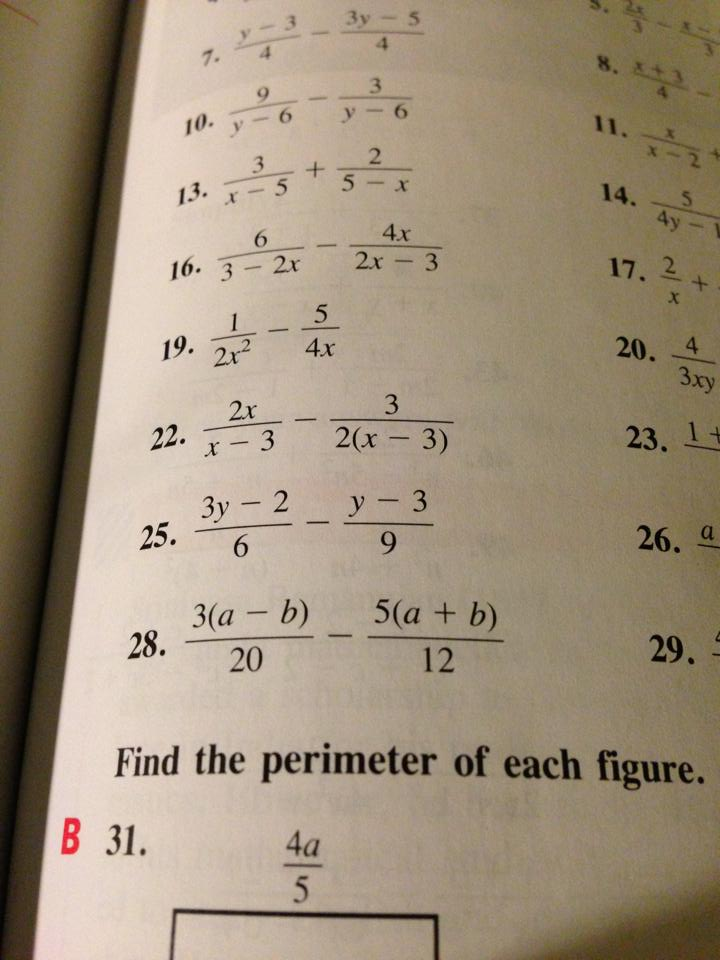
Solved Y 3 4 3y 5 4 9 Y 6 3 Y 6 3 X 5 2 5 X 6 3 2x Chegg Com

Find The Equation Of Image Line And Reflected Line Of 2x 3y 5 0

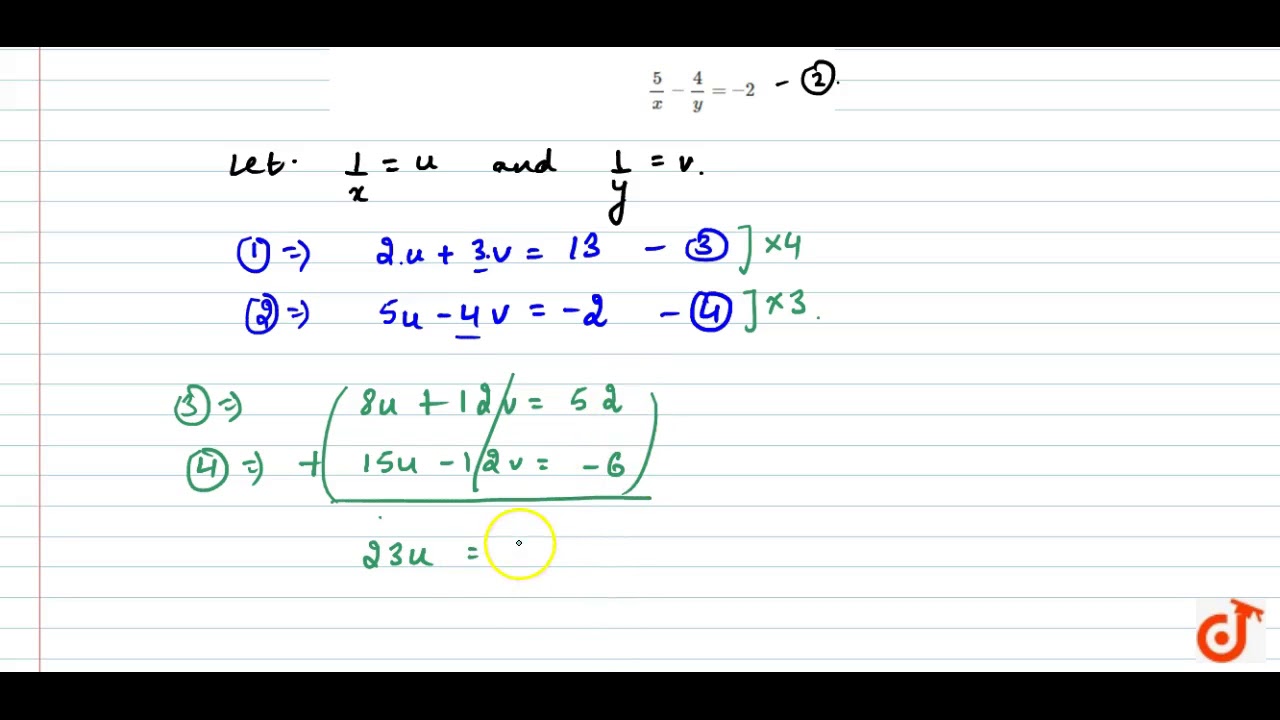
Solve The Pair Of Equations 2 X 3 Y 13 5 X 4 Y 2 Youtube

Transform The Following Equations Into The Form L1 Lambda L2 0 And Find The Point Of Concurrency Of The Family Of Straight Line Represented By The Equation 2 5k X 3 1 2k Y 2 K 0

Ex 3 2 9 Find X And Y If 2 1 3 0 X Y 0 1 2 Ex 3 2

Consider The Function F X Y Ln X 2 Y 2 3 Compute The Partial Derivatives Of The First And Second Order Mathematics Stack Exchange

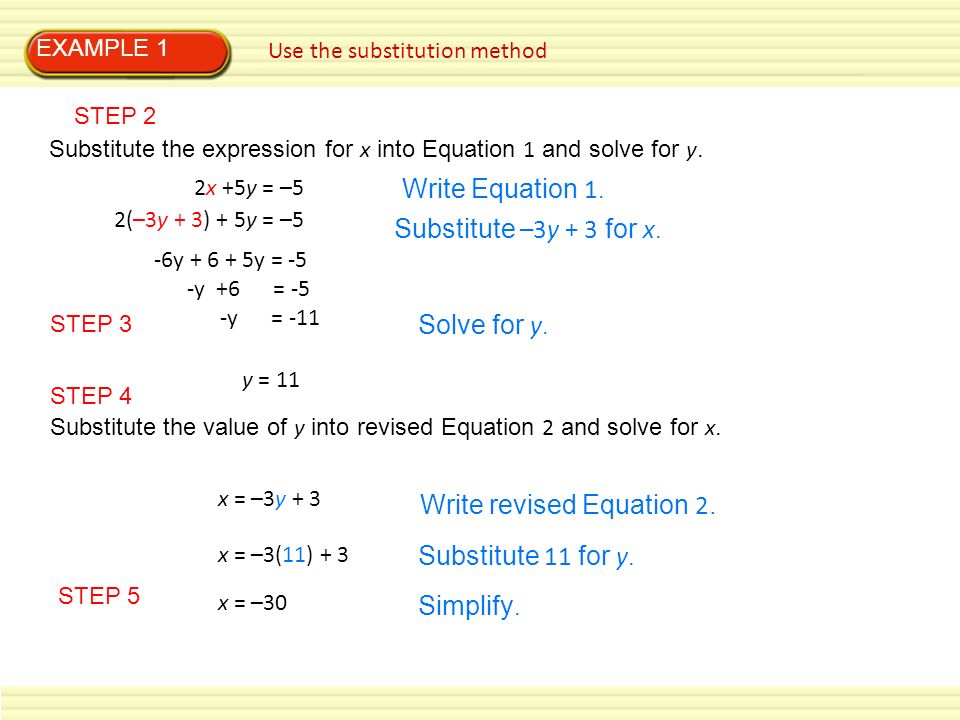
Practice Solving Systems By Graphing 1 2 2x 5y 5 X 3y Ppt Download

Using Matrices Solve The Following System Of Equations 2x 3y 5z 11 3x 2y 4z 5 X Y 2z 3 Sarthaks Econnect Largest Online Education Community

Methods Economics Analysis Final Exam Econ 300 Docsity

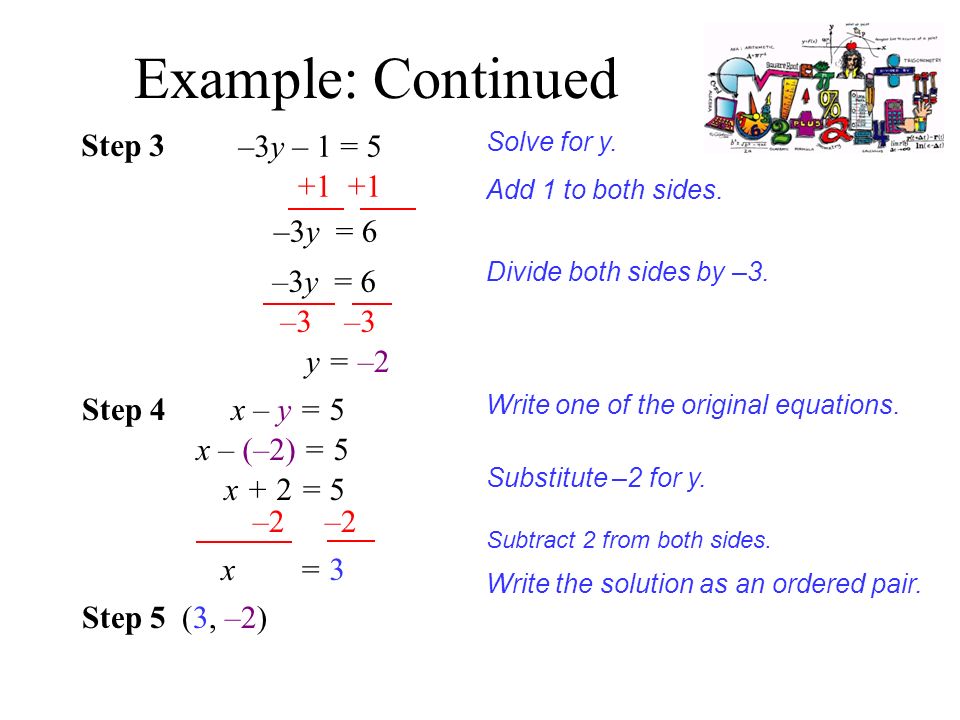
Algebra 2 Solving Systems Algebraically Lesson 3 2 Part Ppt Download

3 Consider The Two Planes P And P2 Where Pi Is Given By The General Equation 2x Y 2 5 And P2 Passes Through The Points 0 0 1 3 2 4 And 2 4 5 A Find L

For Which Values Of A And B Does The Pair Of Linear Equation Teachoo

2 X 3 3 Y 5 0 5 X 1 4 Y 4 0 Solve It By Substitution Method Brainly In

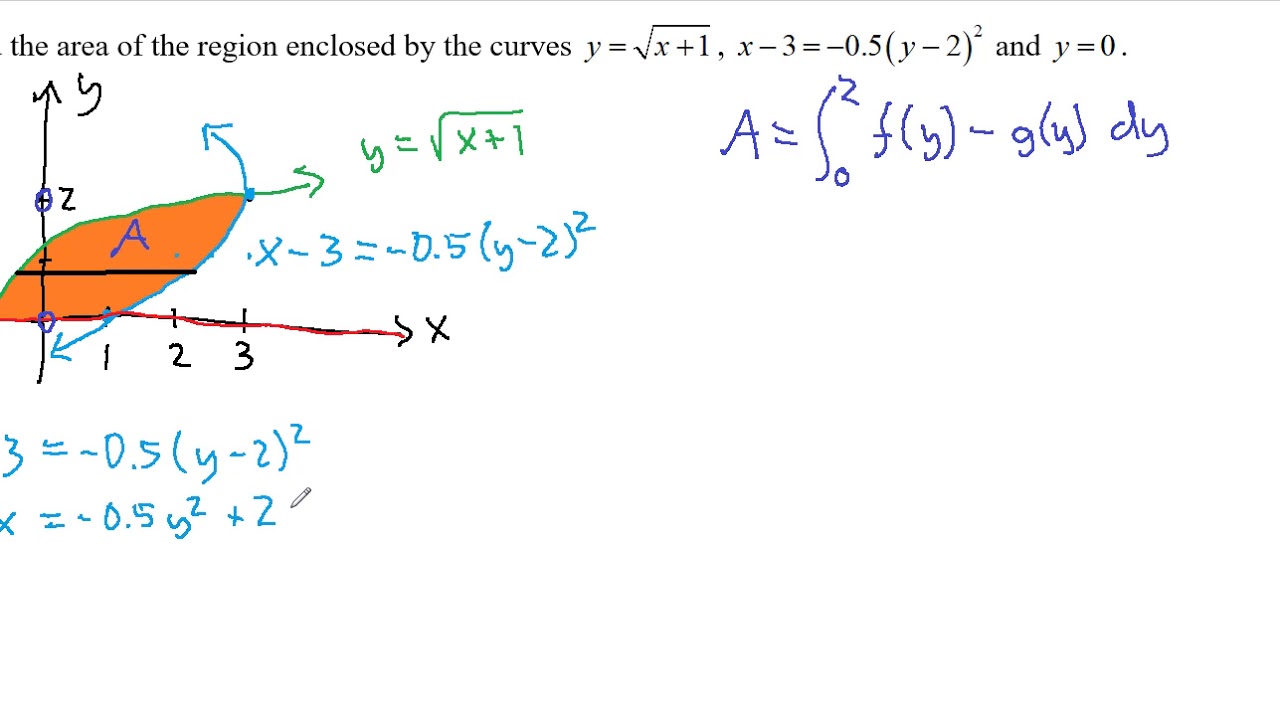
Average Value And Area Revisited

Quadratics Graphing Parabolas Sparknotes

Pyplot Tutorial Matplotlib 3 4 3 Documentation

If 2 X 3y 12 And 3 X 2y 5 Then

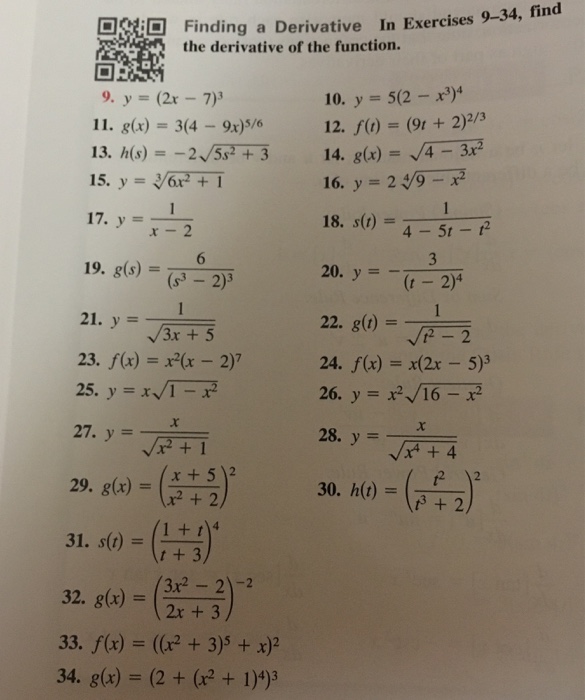
Solved Finding A Derivative In Exercises 9 34 Find The Chegg Com

Answered 17 The Two Points On The Lines 2x 3y Bartleby

Math Chalmers Se